What is Sound?

To grasp why the maximum achievable decibel level is capped at 194, we first need to comprehend the essence of sound itself, which can be described as a form of "vibration." When an object strikes the ground, it produces a loud sound due to the vibrations generated upon impact. These vibrations are transferred through adjacent air particles, propagating outward as sound waves until they reach our ears.

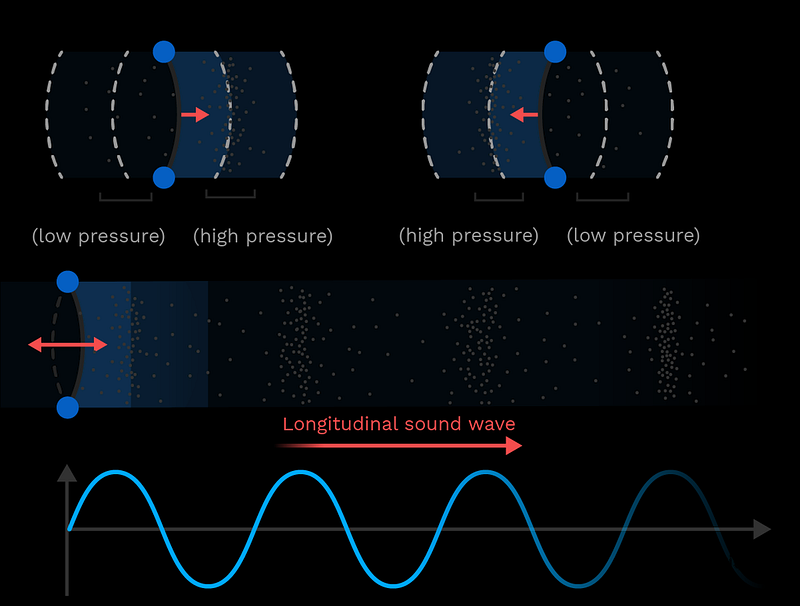
The generation of sound relies on the formation of sound waves—mechanical waves that travel through a medium. The speed at which sound travels depends on the density of that medium. The denser the medium, the more rapidly sound moves, as denser materials have closer particle proximity, facilitating more efficient sound transmission. For instance, sound travels at approximately 340 meters per second in air, around 1500 meters per second in water, and about 5200 meters per second in iron.

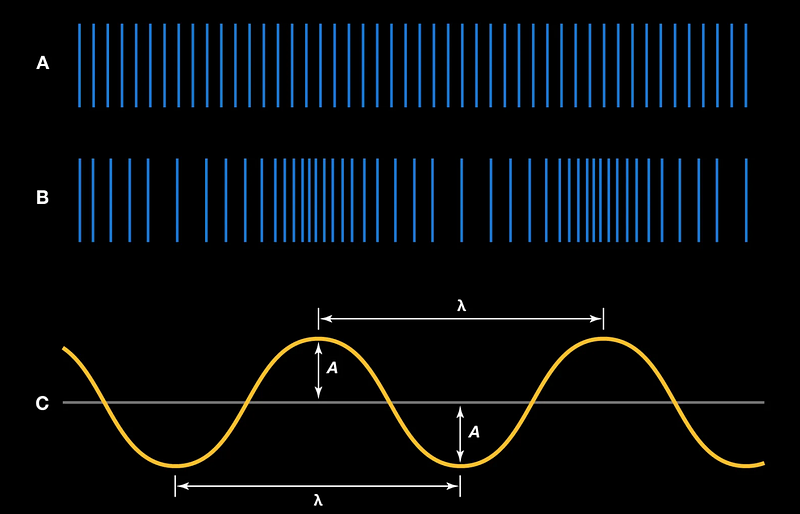
As sound travels, vibrating particles exert pressure on their neighboring particles, resulting in areas of increased pressure known as compression zones. These zones create what is referred to as "sound pressure," typically measured in pascals (Pa). A greater sound pressure corresponds to more energy being transmitted, ultimately resulting in louder sounds. However, in practical terms, sound levels are represented in decibels (dB). But why is this the case?

Why Use Decibels?

Though sound pressure provides a precise measurement of sound intensity, it is often difficult for humans to perceive. For example, a car passing by may create a sound pressure of about 0.2 pascals, whereas a firework explosion can reach 200 pascals. Even though the explosion is significantly louder, the pressure difference is a thousandfold, which doesn't align with human hearing perception.

Thus, the decibel scale is adopted. Decibels are derived from sound pressure measurements, and the correlation between the two is logarithmic. Here’s the formula for converting sound pressure into decibels.

For instance, the sound level of a car is around 80 decibels, while a firework explosion measures approximately 100 decibels. Each increment of 10 decibels signifies a tenfold increase in sound intensity. However, human perception of sound intensity is nonlinear, resulting in considerable variations from actual sound levels. Therefore, a 10-decibel increase roughly doubles perceived loudness. Thus, while a firework explosion has an intensity approximately 20 times greater than that of a car, it only feels about four times louder to our ears.

The 194 Decibel Limit

The decibel scale helps us quantify sound intensity and comprehend how "loud" a sound can be. Here are some common sound levels:

• A gentle breeze rustling leaves: 10 dB
• Flipping pages in a quiet room: 20 dB
• A calm countryside at night: 30 dB
• A quiet urban street: 40 dB
• The sound of a river: 50 dB
• Normal conversation: 60 dB
• An alarm clock: 70 dB
• A motorcycle: 80 dB
• An electric drill: 90 dB
• Fireworks exploding: 100 dB
• An aircraft engine: 140 dB

In day-to-day life, most people encounter sounds peaking around 140 decibels. But is it feasible to produce sounds exceeding this limit?

The answer is no! Sound transmission relies on the vibration of a medium, and when a sound wave's energy reaches its peak, a "sound barrier" effect occurs. This phenomenon arises when the energy of the sound wave is so intense that air molecules collide and compress violently, transitioning from a sparse to a dense state.

This can be likened to a crowd entering a mall; if those at the front move slowly, those behind push forward, creating a bottleneck at the entrance. Once everyone has entered, the surrounding area becomes empty, akin to a vacuum where no air particles exist, making sound transmission impossible.

When individuals attempt to enter a mall but the entrance is too constricted, the build-up of people generates pressure that can shatter glass doors. Similarly, when air particles face immense energy, they accumulate energy and then release it abruptly, resulting in a shock wave. Within this shock wave, a circular "vacuum bubble" forms and expands outward. The greater the energy released, the wider the vacuum bubble's reach, until the energy dissipates and the vacuum state cannot be sustained, allowing sound to propagate again.

Thus, the theoretical decibel level at the center of an atomic bomb explosion is around 200. However, even if one could withstand the force of such an explosion, they wouldn't hear the sound due to the immense energy concentrating into a massive shock wave that obliterates everything in its path.

In reality, the audible sound from an atomic bomb explosion is only perceivable from a distance after the shock wave's energy has diminished, not the actual explosion itself. Scientists believe that the loudest sound recorded on Earth might have been the Tunguska event, which unleashed energy equivalent to 20 million tons of TNT, devastating 2150 square kilometers of forest, reminiscent of an atomic bomb explosion. At the epicenter, humans could not hear anything until the energy dissipated, but individuals 2250 kilometers away were still able to hear the tremendous noise.